A wooden box is released from rest near the top a wooden ramp...

Work and Energy

Introduction

This is word that means a lot of things depending on the context:

Energy Consumption of a Household
Energy Drinks
Auras & Spiritual Energy
Renewable Energy

Energy: basics

James Prescott Joule 1818-1889

Energy is a scalar quantity.

It has SI units of: $\rm kg \cdot m^2/s^2$

The unit is called a Joule, after Mr. Joule

1 Joule = 1 Newton × 1 meter

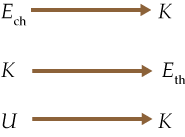
The different energies of a system can be transferred between each other.

Work

Again, we might have word problems here.

For us, Work will be defined as the process of transferring energy between a well-defined system and the environment.

The energy may go from the system to the environment. Or, it may go from the environment to the system

An example of work

A constant force is applied to this box as it moves across the floor.

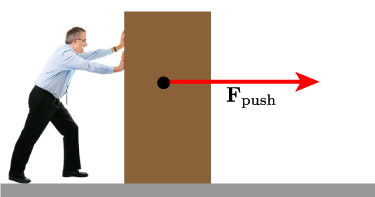
The work done is equal given by: $W = F d$.

This is for the case of a constant force which is applied in the same direction as the box is moving.

If Work is equal to Force times Distance, what are the fundamental SI units of Work?

But what if the force is not in the exact same direction.

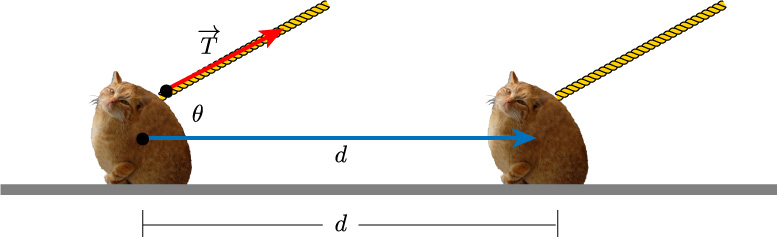
Now we have to consider the component of the force which does point in the direction of the distance traveled.

$$W = F_\parallel d = \mathbf{F} \cdot \mathbf{d} = Fd \cos \theta$$

What is the work being done on the bag by the person carrying it?

I swing a ball around my head at constant speed in a circle with circumference 3 m. What is the work done on the ball by the 10 N tension force in the string during one revolution of the ball?

30 J
20 J
10 J
0.333 J
0 J

Kinetic Energy

We said there we many different forms energy can take (chemical, thermal, potential, etc)

Kinetic Energy is the energy associated with moving objects.

$$\bbox[5px,border:2px solid red]{KE = \frac{1}{2} m v^2}$$

Some example kinetic energies

Ant walking: $1 \times 10^{-8}$ J
Person walking:$ 1 \times 10^{-5}$ J
Bullet: 5000 J
Car @ 100 kph ~ $5 \times 10^5$ J
Fast train ~ $1 \times 10^{10}$ J

Two balls of equal size are dropped from the same height from the roof of a building. One ball has twice the mass of the other. When the balls reach the ground, how do the kinetic energies of the two balls compare? (assume no air resistance)

The lighter one has one fourth as much kinetic energy as the other does.
The lighter one has one half as much kinetic energy as the other does.
The lighter one has the same kinetic energy as the other does.
The lighter one has twice as much kinetic energy as the other does.
The lighter one has four times as much kinetic energy as the other does.

Work and Kinetic Energy

Since the work is related to the amount of energy either entering or leaving a system, we can establish a relationship between the work done on an object and the kinetic energy.

$$W = KE_f - KE_0 \ = \frac{1}{2}mv_\textrm{f}^2 - \frac{1}{2}mv_0^2$$

Potential Energy

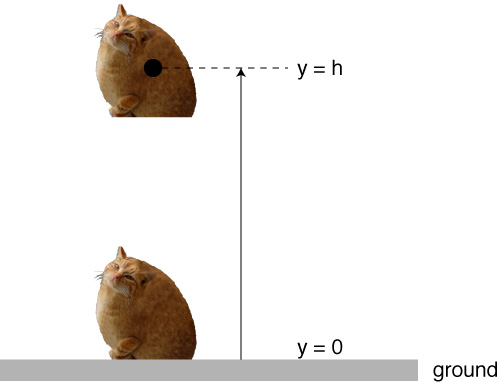
We can store energy in a gravitational system by separating the two objects.

The cat, when lifted off the ground, has a gravitational potential energy.

$$\bbox[10px,border:2px solid red]{U_g = mgh}$$

Potential Energy is only dependent on the height!

It doesn’t matter the path an object takes while climbing higher away from the earth. Any route which ends at the same point will impart the same amount of gravitational potential energy to the object.

Work and Potential Energy

Changing the potential energy of an object requires work.

$$\Delta U = -W $$

In the case of gravity:

"The change in the gravitational potential energy of an object is equal to the negative of the work done by the gravitational force"

$$\Delta U_G = -W_G $$

Gravity does positive work

In this case we let an object drop, and gravity does positive work.

Gravity does negative work

In this case, we raise an object, and gravity does negative work.

Work - Energy

Let's now take a look at systems which have both kinetic and potential energy.

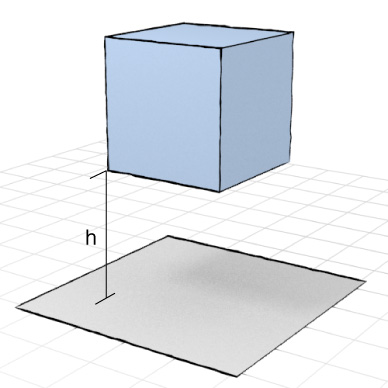
For example: this box which is located at a distance $h$ above the ground.

Usually, we'll call the ground $h = 0$.

If these are the only two types of energy in the system, then we have a very special situation.

We can define the total mechanical energy energy of the system as the kinetic + potential.

$$E_\textrm{mechanical} = KE + U$$

Conservation of Mechanical Energy

If we don't worry about any of those retarding forces like friction or air resistance, then we can say that the total mechanical energy of a system remains the same as time advances.

$$E^\textrm{mech}_i = E^\textrm{mech}_f $$

$$\frac{1}{2}mv_i^2 + mgh_i = \frac{1}{2}mv_f^2 + mgh_f $$

Upon dropping a ball, the total mechanical energy is conserved.

$$E_\textrm{mech} = \textrm{constant} = KE + U_\textrm{grav}$$

Knowing this conservation law, we can use either $h$ to find $v$, or $v$ to find $h$.

The block starts from rest at point 1. Using the conservation of mechanical energy, determine the speed of the block at point 2.

$v = (2gh)^2$
$v = \frac{\sqrt{gh}}{2m}$
$v = \sqrt{2gh}$
$ v = 0$

The block starts from rest at point 1. Using the conservation of mechanical energy, determine the speed of the block at point 2.

$v = (4gh)^2$
$v = \frac{\sqrt{gh}}{4m}$
$v = \sqrt{2gh}$
$v = \sqrt{4gh}$

The block starts from rest at point 1. Using the conservation of mechanical energy, determine the speed of the block at point 2.

$v = (4gh)^2$
$v = \frac{\sqrt{gh}}{4m}$
$v = \sqrt{2gh}$
$v = \sqrt{4gh}$

A car on a hill.

Should this student be worried?

Yes
No
A little bit

A mass $m$ is released from rest on a spherical, frictionless bowl...

If $L$ = 4 meters, how high is the ball? That is, find the distance $h$.

Block on a ramp

Here's a 5 kg block on a ramp, where $\theta = 25^\circ$, and h = 20 meters. Find the work done by gravity and the final speed.

We want to calculate the work done by gravity: $$W = \mathbf{F}_G \cdot \mathbf{d}$$ So, we need to decompose the two vectors into components. Let's call the horizontal direction +x to the left, and the vertical +y up. Thus, in unit vector notion, the force of gravity will be: $$\mathbf{F}_G = - m g\mathbf{\hat{j}}$$ and the displacement will be: $$\mathbf{d} = \frac{h}{\tan \theta}\mathbf{\hat{i}} - h\mathbf{\hat{j}}$$

Now, we can execute the dot product using unit vectors: $$W = \mathbf{F}_G \cdot \mathbf{d} = \left[- m g\mathbf{\hat{j}} \right] \cdot \left[ \frac{h}{\tan \theta}\mathbf{\hat{i}} - h\mathbf{\hat{j}} \right]$$ Since $\mathbf{\hat{i}} \cdot \mathbf{\hat{i}} = 1$ and $\mathbf{\hat{i}} \cdot \mathbf{\hat{j}} = 0$ this dot product simplifies to: $$W = mg h$$ which will be the work done by gravity. Notice how that is same as if we just let the box fall from a distance $h$ above the ground. This situation occurs because the force of gravity is a conservative force, i.e. it is path independent.

To find the speed at the bottom of the ramp, we can use the work-energy theorem which relates the work done to the change in kinetic energy: $$\Delta {KE} = \textrm{Work done}$$ Initially, the box is at rest, so there is no kinetic energy: $$KE_i = 0$$ The kinetic energy at the bottom of the ramp will be given by: $$KE_f = \frac{1}{2}m v^2$$ Thus, the $\Delta {KE}$ will be $\frac{1}{2}m v^2$ Setting this equal to the work done that we calculated above: $$mg h = \frac{1}{2}m v^2$$ allows us to solve for $v$: $$v = \sqrt{2 g h}$$ This result should look familiar.

Notice: we use regular, non-rotated coordinates for this problem. Try it with rotated axes and see what you get. Should it be different?

Ex: The Loop the Loop

We saw that if the velocity of the object was large enough, then it would remain inside in contact with the loop.

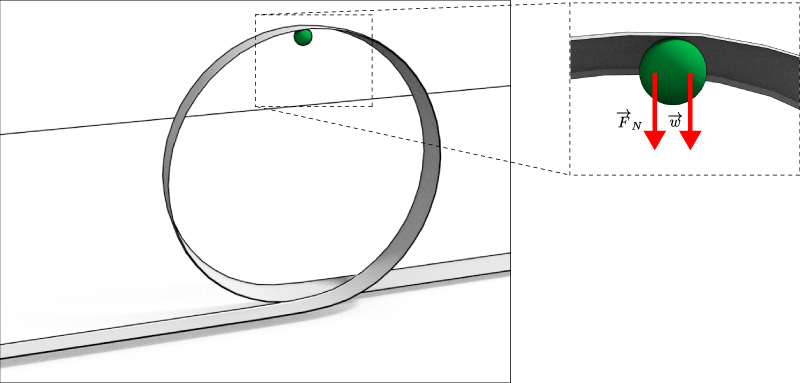
A successful loop-the-loop looks like this. The ball remains in physical contact with the road at all times. (This is another way of saying the normal force is not zero.)

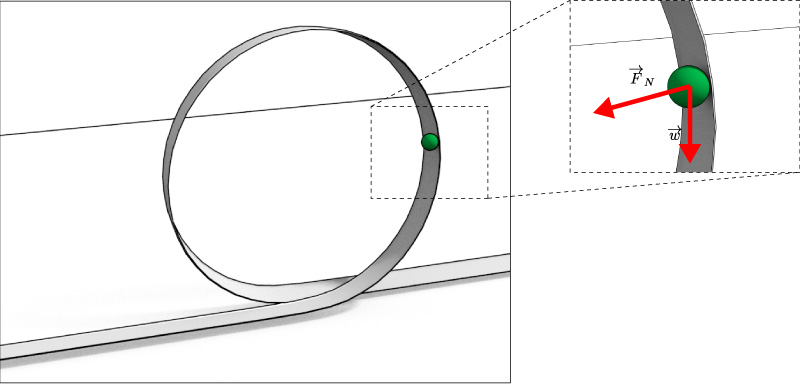
An unsuccessful loop-the-loop looks like this. The ball looses physical contact with the road at all times. (This is another way of saying the normal force becomes zero.)

In the case where the velocity is not fast enough to get the ball all the way around the loop, the ball looses contact with the road near the top.

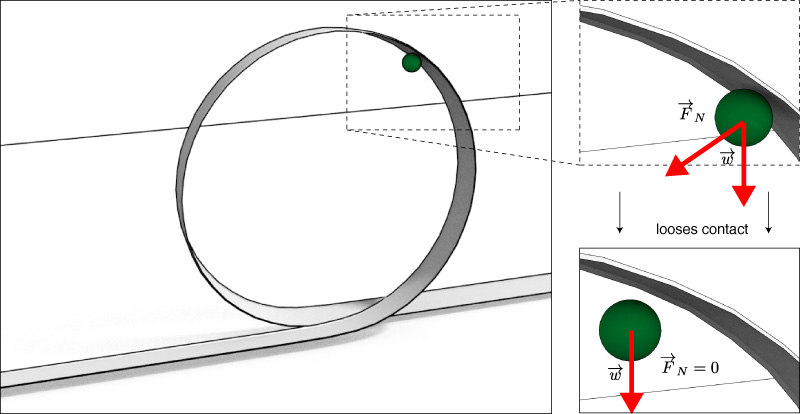
At the top of the loop, the sum of forces pointing towards the ground will be given by:

$$\sum{F}_\textrm{ground} = w + F_\textrm{N} = ma $$

Since this is circular motion, we have

$$ma = m \frac{v^2}{r}$$

Thus we can write:

$$\underbrace{mg}_\textrm{weight} + F_N = m\frac{v^2}{r}$$

What is the minimum velocity at the top of the loop needed to complete the loop-the-loop for a regular, no friction, sliding object.

$v > \sqrt{rg}$
$v > (rg)^2$
$v > \sqrt{\frac{r}{g}}$
$v > \infty$
$v > \sqrt{2rg}$

What is an expression for $h$ that would lead to a speed so the ball maintains contact at all times?

Conservative and Non-conservative Forces

Most of the forces we have seen can be classified as non-conservative.

The exception is Gravity.

Here are two definitions for a conservative force:

1. A force is conservative if the work it does to move an object from point A to point B does not depend on the route chosen (aka the path) to get from point A to point B.

2. A force is conservative if there is zero net work done while moving an object around a closed loop, that is, around a path that has the same point for the beginning and the end.

Examples of Conservative Forces

Gravitational force
Elastic spring force
Electric force

and Non-conservative forces:

Static and kinetic frictional forces
Air resistance
Tension
Normal force

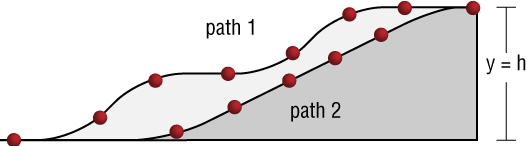
Shown are two paths that a box can take to get from point A to point B

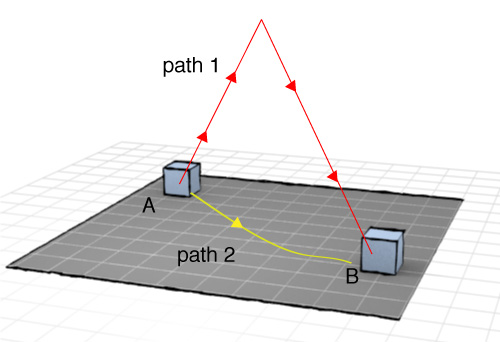
If we look at the change in potential energy, $\Delta U_\textrm{grav}$, then we'll see that the Work done by gravity on the box in either case is equal to zero. And, for any other path you can imagine, it's also zero.

$$W = -\Delta U_G = mg(h-h) = 0$$

Here is an example of a conservative force interacting with a box. If we slide the box from point A to B along path 1, we can calculate the work done by figuring out the work done by gravity to go from the ground up to the apex, and then back down to the ground. Adding these two works together will result in zero, since on the way up, gravity is pointing against the displacement vector: [$F_G d \cos(180^\circ$)] and on the way down, gravity will be in the same direction as the displacement vector: [$F_G d \cos(0^\circ$]. Thus, these two values are equal in magnitude but opposite in sign and so when that are added together will be equal to zero. In the case of path 2, the work done by gravity will be zero also, since there is no change in height along the path. Thus, no matter how we chose to go from the A to B, the net work done by gravity will be zero. This is the definition of a conservative force.

Moving a box along 2 paths in the presence of friction.

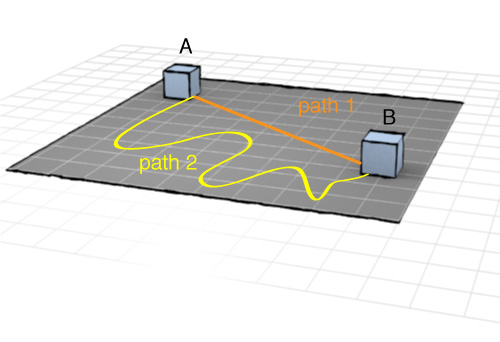
The situation is very different if we ask about the work done against friction during these two paths. Path 2 will require more work than path 1.

And so, forces like friction and air resistance are considered non-conservative.

We can however account for these forces in our analysis of certain systems.

$$W_\textrm{NC} = E_f - E_0$$

If the change in energy is non-zero, bewteen the initial and final times of a mechanical system, then there must be some non-conservative forces doing work during the motion.

Now, we have a ramp that has some friction.

How can we describe the motion of a box on this ramp?

A child, starting from rest, slides down a slide. On the way down, a kinetic frictional force (a nonconservative force) acts on her. The child has a mass of 50.0 kg, and the height of the slide is 18 m. If the kinetic frictional force does $6.50 \times 10^3$ Joules of work, how fast is the child going at the bottom of the slide?

If the object slides down a hill with an elevation of 10 m, where will it stop? Consider the sloped part frictionless and the rough part at the bottom having a $\mu_k$ equal to 0.3.

Power

Again, here's another word we have to be very careful with. We might be used to calling anything that seems strong 'more powerful'.

For us however, we'll need to restrict our use of the word power to describe how fast a process converts energy.

$$\textrm{Power} = P = \frac{\textrm{change in energy}}{\textrm{time}}$$

The SI unit of power is called the watt: $$ \rm 1 watt = 1 joule / second = 1 kg \cdot m^2 / s^3$$

A unit of power in the US Customary system is horsepower:
1 hp = 746 W

Units of [power - time] can also be used to express energy (e.g. kilowatt-hour) $$1 \rm kWh = (1000 W)(3600 s) = 3.6 \times 10^6 J$$

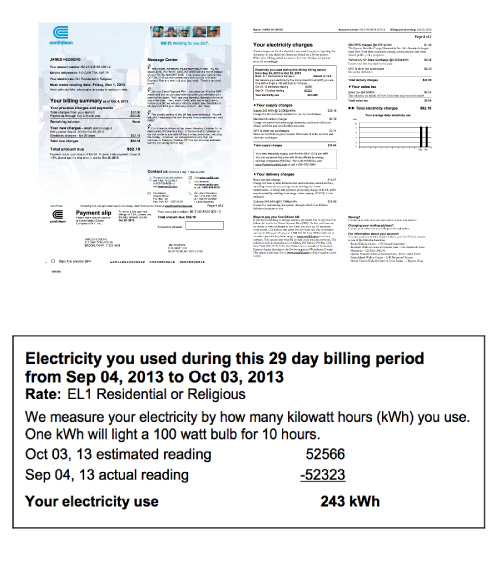
We should be able to understand these mysterious documents now.

How many Joules of energy did I use?

How many pushups would I have to do to generate that much energy?

I used to work out on a rowing machine. Each time I pulled on the rowing bar (which simulates the oars), it moved a distance of 1.1 m in a time of 1.6 s. There was a little display that showed my power and it said 90 Watts. How large was the force that I applied to the rowing bar?

A go kart weighs 1000 kg and accelerates at 4 m/s² for 4 seconds. What is the average power?

Variable and Constant Forces

In the most basic situation, the force applied to an object was constant. When this is the case, the Work was given by:

$$W = \mathbf{F} \cdot \mathbf{d}$$

This is also equal to the "area under the curve"

Work done by a variable force

However, it's also possible that the work will vary as a function of distance. In this case, the work will not be given by the standard form, because, the F is not constant.

$$W \neq \mathbf{F} \cdot \mathbf{d}$$

However, the work is still equal to the "area under the curve"

Work done by a variable force

We don't have the tools (i.e. integration) to be able to do much with this at this level of physics.

Work done by a variable force

To deal with these situations, we'll have to use some integration techniques.

Here's an applied force that varies as the square of the distance.

We could figure out the area under the curve, which does equal the work, but performing the integral

$$W = \int_{x_i}^{x_f} F(x) dx$$

In this case:

$$W = \int_{x_i}^{x_f} x^2 dx = \frac{1}{3}x^3\biggr|_{x_i}^{x_f}$$

In the case of 3d:

If our forces are variable and 3 dimensional, (and perhaps not pointed directly in the direction of the displacement), then we have to use some more developed vector calc:

$$dW = \mathbf{F} \cdot d\mathbf{r} = F_x dx + F_y dy +F_z dz$$

Or, if we integrate over the distance:

$$ W = \int_{r_i}^{r_f} dW = \int_{x_i}^{x_f} F_x dx + \int_{y_i}^{y_f} F_y dy + \int_{z_i}^{z_f} F_z dz $$

Since work is a scalar, then these terms will just add up without any complications.

Recover the work-kinetic energy equation?

$$W = \int_{x_i}^{x_f} F(x) dx$$

We should be able to show that this gets back to kinetic energy somehow...

Based on the above:

$$\Delta U(x) = -W = -F \Delta x$$

Now we can take the derivative with respect to x:

$$\bbox[10px ,border:3px solid red]{F(x) = -\frac{dU(x)}{dx}}$$

Based on the the relation between work and potential energy:

$$\Delta U(x) = -W = -F \Delta x$$

Let's rearrange

$$\bbox[10px ,border:3px solid red]{F(x) = -\frac{\Delta U}{\Delta x}}$$

Draw plots of the gravitational potential energy and the gravitational force for an object as a function of height above the earth. Consider only small distances above the surface of the earth.

If the force from an elastic band when stretched is given by $F = -k x$, what would the change in potential energy be for an object attached to the rubber band and pulled back by a distance d? (k is a constant that is determined by the rubber band material ) Plot the force and $U_\textrm{rubber band}$ as functions of distance.

Shown is the potential energy of an unknown force as a function of distance along the x-axis. Where would that force be the largest, and pointed in the positive direction?

A diatomic molecule has two atoms (H₂ for example). If the potential energy of such a system is given by:

$$U = \frac{A}{r^{12}} - \frac{B}{r^6}$$

Find the equilibrium separation of such a molecule. That is, find a distance $r$ that will lead to zero net force on each atom.(A and B are just constants)

Here are plots of the Potential function, and its derivative (i.e. force).

$$U = \frac{1}{r^{12}}-\frac{1}{r^6}$$

Force Microscopy

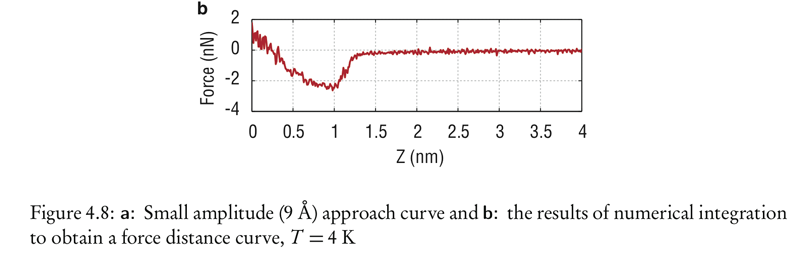
More info: Intro to AFM

Emmy

23 March 1882 – 14 April 1935

Emmy Noether was a theoretical physicist who did pioneering work in the study of conservation principles.