Light & Matter Interactions
Spectral Lines
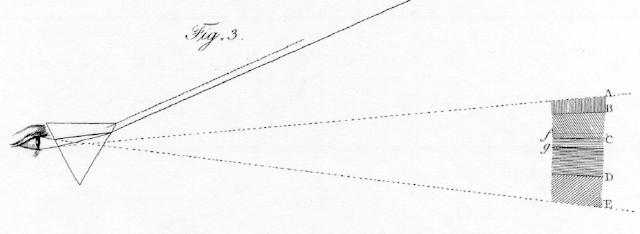
William Hyde Wollaston [1766 - 1828] generated a solar spectrum using a prism, and noticed black lines in some sections. "The line A that bounds the red side of the spectrum is somewhat confused, which seems in part owing to want of power in the eye to converge red light. The line B, between red and green, in a certain position of the prism, is perfectly distinct; so also are D and E, the two limits of violet. But C, the limit of green and blue, is not so clearly marked as the rest; and there are also, on each side of this limit, other distinct dark lines, / and g, either of which, in an_ imperfect experiment, might be mistaken for the boundary of these colours."
Reproduced from Philosophical Transactions of the Royal Society of London, vol. 92 (1802), p. 380 (Plate XIV).
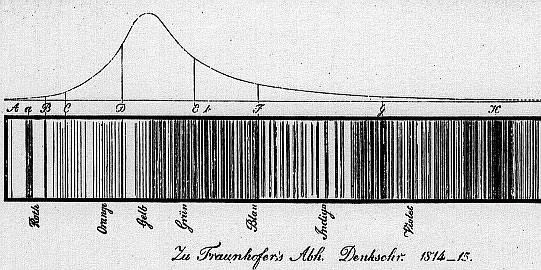
Joseph con Fraunhofer [1787-1826] rediscovered the lines 15 years after Wollaston. If the physical origins of the lines were understood, then we could learn more about the sun and what makes it work.
Denkschriften der K. Acad. der Wissenschaften zu München 1814-15, pp. 193-226.
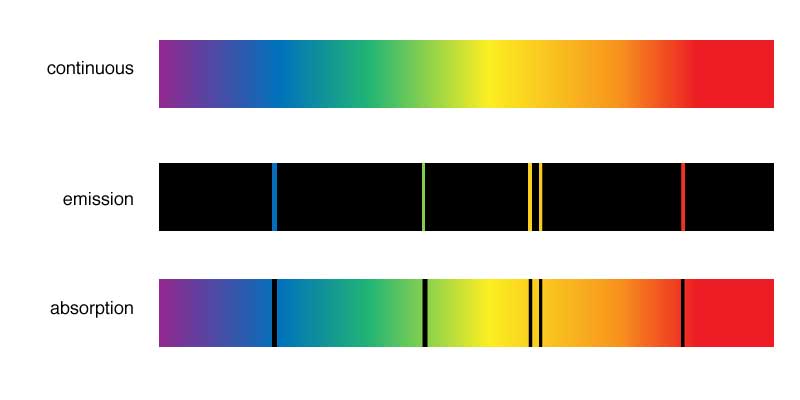
Different types of spectra: continuous, emission, and adsorption.
Kirchoff's Laws
- A hot, dense gas or hot solid object produces a continuous spectrum with no dark spectral lines
- A hot, diffuse gas produces bright spectral lines (emission lines)
- A cool, diffuse gas in front of a source of a continuous spectrum produces dark spectral lines (absorption lines) in the continous spectrum.
Inside atoms
$$\begin{equation} \frac{1}{\lambda} = R_H \left( \frac{1}{m^2} - \frac{1}{n^2} \right) \end{equation}$$
If we let $m = 2$ in the above equation, then setting $n = 3,4,\ldots,6$ yields the following wavelengths for the expected emissions.
| n | $\lambda$ [nm] |
|---|---|
| 3 | 656.3 |
| 4 | 486.1 |
| 5 | 434.0 |
| 6 | 410.2 |
| 7 | 397.0 |
Classical Atoms
According to Maxwell's laws of EM, a charge moving in a circle should radiate energy because of the centripetal acceleration.
The Bohr Model
The Bohr Radius
Starting with Coulomb's Law and $F = ma$: $$\begin{equation} -\frac{(Ze)e}{4 \pi \epsilon_0 r^2} = - \frac{m_e v^2}{r} \end{equation}$$ Then taking the kinetic energy of the orbiting particle: $$\begin{equation} K = \frac{1}{2}m_e v^2 = \frac{Ze^2}{8 \pi \epsilon_0 r} \label{eq:kineticenergyelectron} \end{equation}$$ The potential energy is $$\begin{equation} U = - \frac{Ze^2}{4 \pi \epsilon_0 r} \end{equation}$$ Summing the kinetic and potential to get the total energy: $$\begin{equation} E = K + U = \frac{Ze^2}{8 \pi \epsilon_0 r}-\frac{Ze^2}{4 \pi \epsilon_0 r} = -\frac{Ze^2}{8 \pi \epsilon_0 r} \end{equation}$$
The Bohr model suggests that instead of the total energy being capable of taking on any value, instead it can only take on quantized integer multiples of a constant value. Or, in terms of the angular momentum: $$\begin{equation} L = m_e v r = \frac{n h}{2 \pi} = n \hbar \end{equation}$$ Solving for $v$ and combining this with the kinetic energy from equation \eqref{eq:kineticenergyelectron} we'll obtain for the radius, $r$: $$\begin{equation} r_n = \frac{4 \pi \epsilon_0 \hbar^2}{Z e^2 m_e} n^2 = \frac{5.29 \times 10^{-11} \; \textrm{m}}{Z}n^2 \end{equation}$$ Here, $n$ is called a quantum number and can take integer values starting with 1. $Z$ is the number of protons in the nucleus. If $n = 1$ and $Z = 1$, which corresponds to the lowest energy level of the hydrogen atom, $$\begin{equation} r_\textrm{Bohr} =0.529 \times 10^{-11} \; \textrm{m} = 0.529 \unicode{x212B} \end{equation}$$ ($1 \unicode{x212B} = $ one tenth of a nanometer or $1 \times 10^{-10} \; \textrm{m}$)
Lowest energy
The energy levels of the hydrogen atom.
$E_1$ is the lowest energy of the hydrogen atom: -13.6 eV.
Now, if we are interested in the difference between two energies levels of an electron: $$\begin{equation} \Delta E = E_\rm{high} - E_\rm{low} = E_\rm{photon} \end{equation}$$
Spectrum Lines
Find the wavelength of a photon emitted during a transition from $n = 3$ to $n=2$ for an electron in a hydrogen atom.
Kirchoff's laws, again
- A hot gas, or hot solid produces blackbody radiation. This is a continuous spectrum that follows Plancks Law for $B_\lambda(T)$.
- A hot diffuse gas produces emission lines caused by energy lost by electrons as they go from a higher level to a lower level and emit photons at the corresponding frequencues.
- A cool diffuse gas in front of a source of continuous spectrum will have dark lines when an electron gets excited by incoming energy.
Quantum Theory
The Photoelectric Effect
Photons
The energy of a single, reddish, photon: $$\begin{equation} E_\textrm{photon} = h\nu = \frac{hc}{\lambda} \simeq \frac{1240 \; \textrm{eV nm}}{700 \; \textrm{nm}} = 1.77 \; \textrm{eV} \end{equation}$$ is about half that of a blue photon: $$\begin{equation} E_\textrm{photon} = h\nu = \frac{hc}{\lambda} \simeq \frac{1240 \; \textrm{eV nm}}{400 \; \textrm{nm}} = 3.10 \; \textrm{eV} \end{equation}$$
de Broglie wavelength
$$\begin{equation} \lambda = \frac{h}{p} \end{equation}$$Uncertainty Principle
$$\begin{equation} \Delta x \Delta p \ge \frac{1}{2}\hbar \end{equation}$$Other forms of the uncertainty Principle
$$\begin{equation} \Delta E \Delta t \approx \hbar \end{equation}$$Tunneling
Tunneling through a barrier.